|
This page represents only my own views, and not those of any university or other body. Posted Thursday 22nd May 2014 at 11.06am PAM v BRW 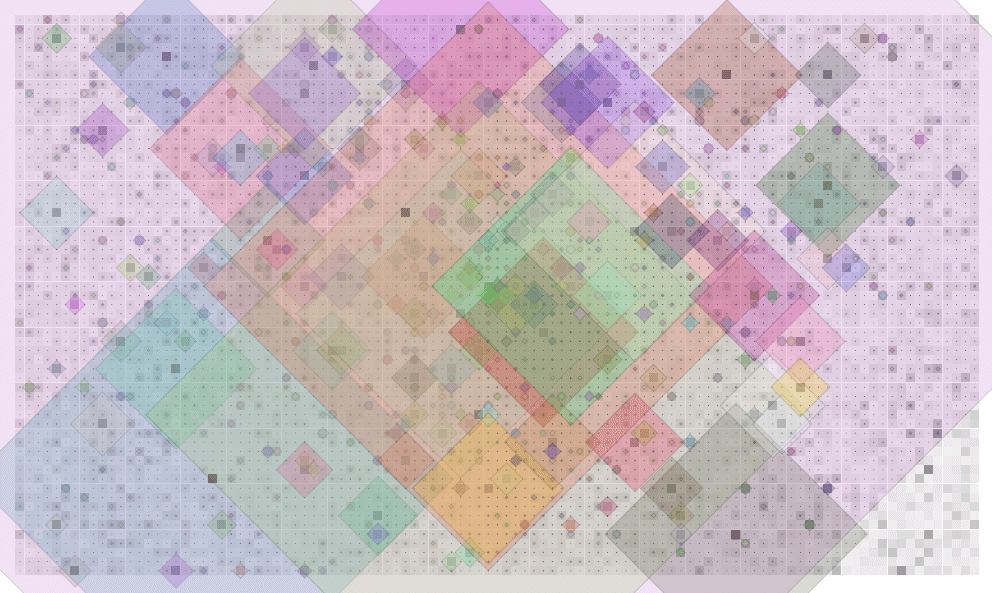 Marcel Ortgiese and I have just uploaded a paper to the arXiv. The model we investigate is as follows: start with a single particle at the origin. Each particle performs a continuous-time nearest-neighbour symmetric random walk on Zd. When at site z, a particle splits into two new particles at rate ξ(z), where the potential ξ(z), z in Zd is a collection of non-negative i.i.d. random variables. The two new particles then repeat the stochastic behaviour of their parent, started from $z$. So we end up with lots of particles spread across a region of Zd, and the question is where these particles are located. There's a battle between the random walk kernel, which wants to spread everything out, and the potential, which wants some points to have more particles than others. Until now most of the analysis has looked at the expected number of particles, also known as the Parabolic Anderson model. We look at the actual number of particles, and things get really interesting when the potential is heavy-tailed, specifically when P(ξ(z)>x) = x-α for x>1 and some fixed α>d. We show that things essentially look like a system of growing "lilypads" (unfortunately these are L1 lilypads, so they look like diamonds, not circles). You can see the lilypads in Z2 in this simulation. Be patient! Return to blog Comments Write a new comment: |