Accessible maths at university
From retrofitting to inclusive design?
Emma Cliffe
Plan
The hidden transition
Maths at university
"Generally, students are surprised by both the teaching method at university and the nature of university mathematics"
Thomlinson, Challis, and Robinson. 2009. Student Experiences of the Transition to University. MSOR Connections 9 (2): 48–51.
| School | University |
|---|---|
| Characterised by learning of processes through 'doing'; mastery in class possible | Characterised by learning of concepts; mastery in class unusual |
| Directed learning; repetitive practise; regular feedback | Independent learning; explorative practise |
| Can clarify in 'real time' | Often can't clarify in class |
| Maths resources provided in required formats | Expected to access maths resources independently |
| Specialist support near level of mathematical fluency | Specialist support with maths background rare |
Mathematics at university level is
- "characterised by the need for a high degree of conceptual and abstract thinking within the learning process"
We need students to have the processing capacity to engage with concepts and abstract thinking.
- Students with a print disability may spend substantial processing capacity on entirely different tasks.
"as the mathematics advanced he came across symbols he didn’t recognise and had trouble relating [...] lectures to his notes, or reading aloud his work for a scribe"
[one lecturer] "did read out everything and the equations just got too long and I lost concentration half way through and I couldn't keep track."
"first, it’s hard to follow a long equation, even if it’s read out, and second, they don't always remember, which I don't blame them. But even if they read it out I don’t think it's gonna be that helpful, [...] a page long of proof which even if they read it out you're not gonna follow in your head."
Rowlett & Rowlett, 2012. Experiences of students with visual impairments in Good practice on inclusive curricula in the mathematical sciences.
"[maths is by] nature nonlinear [...] As severely VI people, we [are] confined to work in a one-dimensional space."
"acquiring properly translated Braille is a highly complicated task [... we developed] script files to process [...] LaTeX"
"The most important reading materials to be obtained are lecture notes. [... We] follow the lecture by referring to the notes on a laptop computer, rather than having to spend time catching up afterwards."
"producing Braille [...] and relying on a transcriber [...] limit[s] the student's independence of study and collaboration with their peers. We strongly believe in the use of LaTeX [..]"
Williams & Irving, 2012. On the accessibility of mathematics to visually impaired students in higher education.
"[We] developed [our] working methods on an ad-hoc basis as necessary, guided at younger ages by [our maths teacher] David Spybey"
"By employing the methods documented here both authors achieved class I Masters mathematics degrees"
Williams & Irving, 2012. On the accessibility of mathematics to visually impaired students in higher education.
"Learning to use the A&R method is an 'extra' for students whether it is learnt alongside A levels or later but it brings with it a level of independence that should be the right of any blind student."
Spybey, 2012. Mathematics for visually impaired students at A-level and the transition to degree level.
Key points
- Applicants may make assumptions about the university mathematics learning environment.
- Successful methods of working with school level mathematics do not necessarily scale or carry over the hidden transition.
- The increasing symbol set is difficult if written and spoken formats don't align in context.
- Inappropriate linearisation of higher level maths places too high a cognitive load.
- Students are best placed if their working method is compatible with how mathematicians write so, LaTeX.
- Input from a maths access specialist can enable a substantial level of independence but this skillset is rare.
Retrofitting

A 15 year journey... in maths and computer science
Quality Assurance Agency for HE (2015)
Learners benefit from seeing arguments developed [...] in 'real time' [...] Traditional board-based lectures continue to be widely used, often augmented by more interactive teaching approaches.
Equality and Human Rights commission (2011)
A common anticipatory adjustment of providing resources in advance in electronic formats.
What we want...
For all students to follow the lecture and to engage with the concepts and arguments!
We don't want other tasks taking precedence or lost students.
- "[I am] struggling to make accurate notes during the lecture."
- "Without the lecture notes there is no point in being there"
- "The notes our lecturer uploads [...] are in hand-written format which I find extremely difficult to read"
- "My eyes don't especially like the font and it is all a bit close together."
- "I often find my eye wanders around the page with some of the fonts that are used making it really difficult to follow what is being said/asked"
- "I wondered if I could get some editable ones as theres lots of gaps and font size changes on the PDFs online which make it really hard when I'm trying to read them."
- "Speech doesn't work..."
- "I would like to highlight that pdf files are not useful for me"
- 2004-7: Blind student (CS), invented working methods
- 2005/6: Blind student (Maths), specially produced notes
- 2006/7: Two partially sighted students (Maths), specially produced notes; other disabled students offered these
- 2007-present: Only one academic year in which we had no maths student with visual impairment
- 2010/11: Interpretation of "anticipatory measures"
- 2011/12: HESTEM, fragile single master & conversions
- 2012: Student declares VI during term, notes within day!
- 2015/16: Substantial changes to technology; produced single master more robust to conversion
- 2015-18: Blind student (CS), supported and empowered via informed lecturers and general maths support staff
- 2016/17: Some lecturers (Maths) convert own notes
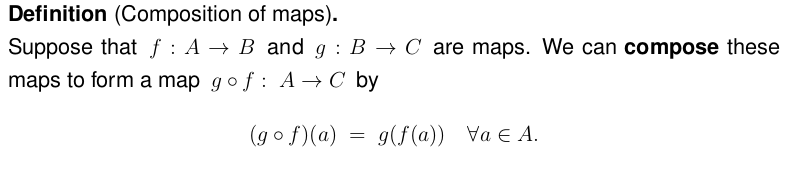
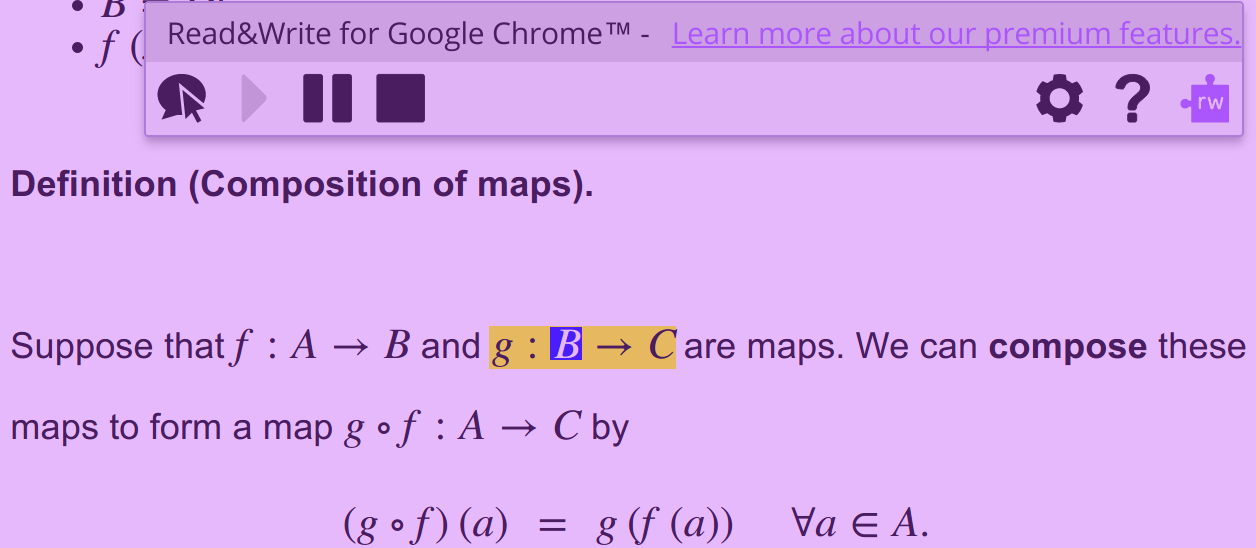
- "The combination of notes I have prior to lectures and the narrative that lecturer add, enables me to determine words and equations written on the board."
- "I have been finding them much easier to read compared to other resources"
- "[It helps to] have notes and handouts laid out electronically, well-spaced out and so I can search within them using my computer"
- "The clear print notes are incredibly useful."
- "The LaTeX is verbose and contains layout commands I do not need."; "This ['human readable' LaTeX] is better."
- "I would just like to say thank you. This service has been a great help over the last few years. Thank you very much for organising it and for providing quick help when it was needed."
From retrofitting...
I have spent the last 15 years trying to retrofit accessible interfaces to the entirely natural community evolved handwritten, typeset and old software based maths learning environments. It is important to understand that this is a side effect of a more general difficulty - mathematicians continue to hand write and then, maybe, typeset for a reason!
But, retrofitting is an expensive, inefficient compromise reliant on rare skill sets, research output organically grown tools and, to be honest, serendipity!
We rely on expertise at the intersection of higher level maths, programming and access.
How can this possibly scale?
...to inclusive design?
I am surprised by the rate of change in what is possible
But it is provably impossible to convert general LaTeX/TeX into modern structured formats. Keeping to transformable subsets within unlimited freedom and extensibility is hard. We need to find an acceptable limiting of this freedom.
As the mathematical community move from paper and board to computer can those in the intersection help build environments which guide or limit all mathematicians to create inclusive mathematical learning materials by design?
If we wait until the mathematical community's e-communication tools fully mature it may be too late to act!
Research, development, infrastructure, training
- Know how sighted people consume and produce maths and diagrams including how and why formats evolve
- Enable design of linearised interaction methods which minimise cognitive load.
- Define most expressive LaTeX-variant enabling robust content writing, not typesetting, for multiple renderings.
- Stable building block development e.g. MathJax, Liblouis.
- Autogeneration of alternative interaction for structured diagrams and visualisation e.g. BrailleR, Desmos.
- Map community software progress in building block use to support inclusive design e.g. R/RMarkdown/RStudio
- Enable university procurement e.g. TEL, computing services, librarians to query and test for maths access.
What are we doing in the short term?
- Bath Maths TEL community of practice: Turns out that recruiting a STEM TEL specialist is amazingly helpful and he is bringing together people from across the institution.
- Word: Maths in Word documents training (with TEL).
- Graphs: Drawing a graph? Can Desmos do it? Then use Desmos: we can show you how and why (with TEL).
- E-assessment: Moving to semi-accessible community platforms and getting involved to influence direction.
- RMarkdown: Entice some statisticians from LaTeX to R/RMarkdown/RStudio/BrailleR (Is this good enough? If not, why not? If yes, how would they sell it or something similar to the mathematicians?). I have absolute faith in this plan... TEL is waiting to see what happens!
Questions and discussion
These slides are available at:
http://people.bath.ac.uk/cspehj/slides/MathsUniRetroToInc/
My email address is:
E.H.Cliffe@bath.ac.uk

